One of the ways Dr. Richard Carrier defends his contention that time can arise from a state of absolute nothingness is by giving one example where this may have happened: the singularity at the origin of our universe.
In this post, I will attempt to refute this by arguing that this singularity was not really a state of absolute nothingness at all.
A couple of assumptions
Carrier's example assumes two things that may or may not be true but which I am happy to allow because they appear reasonable and do not pose a problem to my objections.
Firstly, the argument assumes that time itself was created in the Big Bang though this may not in fact be the case. I agree with Carrier, however, that there does not seem to be any obvious reason why it could not be the case for some possible universe (if not this one), and so for this discussion let's assume that time did in fact begin with the Big Bang. That is to say, there was no time prior to the Big Bang.
Carrier's argument
Perhaps it's best to quote from the man himself in order to explain his point.
I agree with Carrier when he says "Clearly it is not logically impossible to have shapes that begin from a singular point of time but consist of more than a singular point of time", at least if we substitute the word "single" for "singular".
This is not my argument however. My argument is not that timelines cannot start with a single point. Instead, I argue that in a state of absolute nothing, there is no timeline! Without a timeline, nothing can happen, including changing a temporal singularity into a timeline.
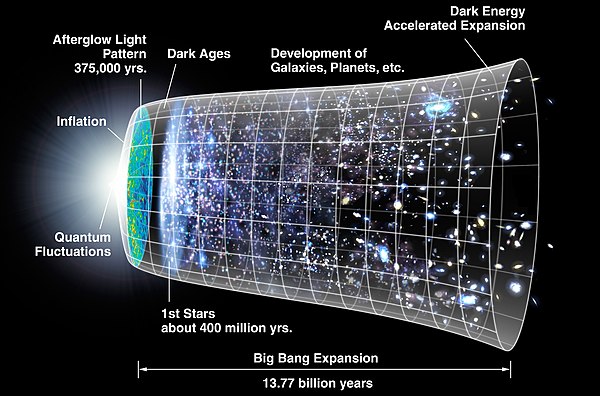
And that word "temporal" is important, because the point of space-time at the big bang was a spatial singularity only. In my view, the collapsed point of space-time that exists in a state of absolute nothingness is something else entirely.
The point of space-time at the Big Bang has at least four dimensions -- there could be even more if the string theorists have their way. Conversely, I would argue that in a state of absolute nothingness, there would be zero dimensions of space and zero dimensions of time. The singular point of space-time in absolute nothingness would therefore not be 4D but 0D.
Furthermore, the singularity of absolute nothingness is both a temporal and a spatial singularity. It contains all of time and all of space within it, because there is no time and space. That of the Big Bang is spatial only, because it does not contain all of time within it. This is why I object to Carrier's description of the Big Bang as a "singular" point of time, and this is why I do not believe that Carrier's analogy applies.
A confusing contradiction
In this post, I will attempt to refute this by arguing that this singularity was not really a state of absolute nothingness at all.
A couple of assumptions
Carrier's example assumes two things that may or may not be true but which I am happy to allow because they appear reasonable and do not pose a problem to my objections.
Firstly, the argument assumes that time itself was created in the Big Bang though this may not in fact be the case. I agree with Carrier, however, that there does not seem to be any obvious reason why it could not be the case for some possible universe (if not this one), and so for this discussion let's assume that time did in fact begin with the Big Bang. That is to say, there was no time prior to the Big Bang.
Secondly, Carrier assumes that a state of absolute nothingness is mathematically equivalent to a single point of collapsed space-time. It is not clear to me that this is so, however I am perfectly content to allow this assumption also.
Carrier's argument
Perhaps it's best to quote from the man himself in order to explain his point.
A better way to think of it is as a problem in non-Euclidean geometry.
Let’s assume we’re only talking about universes with two dimensions of space and one of time. These can be described as a three dimensional shapes (which will be familiar to you).
At one end of which (call it the “Big Bang”) is a singular point of time on the left hand side at which everything begins (from which a volume of space expands). But it’s a static shape (say, a cone, for example), and all time exists along its axis (a world tube for the whole universe). That is one possible universe.
We can use this same process to model a nothingverse (in that case we have only the singular point of time and space and nothing else), and a somethingverse without time (a singular point of time surrounded by a plane of spatial points at which things exist statically, with no change; this could have the shape of a wheel, for example, where the plane of the wheel is the time dimension and two dimensions of space are the points along the wheel), and a somethingverse with time (this would be a solid shape, a volume, one radius of which is the time axis, the rest the two dimensions of space, each slice along the time axis being that 2D space at a different time).
Since all of these shapes are logically possible, all of these universes are logically possible. It would be illogical to point to the starting point of time, that singular point of time all the way to the left, and say no other shapes are possible because those shapes would require transitioning from that one-dimensional point, and that contradicts the fact that transitioning would require more points of time to exist first, but since they don’t, they can’t. Since we can imagine those other shapes existing (instantaneously and thus eternally), this refutes the argument. Clearly it is not logically impossible to have shapes that begin from a singular point of time but consist of more than a singular point of time.Types of singularity
I agree with Carrier when he says "Clearly it is not logically impossible to have shapes that begin from a singular point of time but consist of more than a singular point of time", at least if we substitute the word "single" for "singular".
This is not my argument however. My argument is not that timelines cannot start with a single point. Instead, I argue that in a state of absolute nothing, there is no timeline! Without a timeline, nothing can happen, including changing a temporal singularity into a timeline.
And that word "temporal" is important, because the point of space-time at the big bang was a spatial singularity only. In my view, the collapsed point of space-time that exists in a state of absolute nothingness is something else entirely.
The point of space-time at the Big Bang has at least four dimensions -- there could be even more if the string theorists have their way. Conversely, I would argue that in a state of absolute nothingness, there would be zero dimensions of space and zero dimensions of time. The singular point of space-time in absolute nothingness would therefore not be 4D but 0D.
Furthermore, the singularity of absolute nothingness is both a temporal and a spatial singularity. It contains all of time and all of space within it, because there is no time and space. That of the Big Bang is spatial only, because it does not contain all of time within it. This is why I object to Carrier's description of the Big Bang as a "singular" point of time, and this is why I do not believe that Carrier's analogy applies.
I want to clearly articulate why the single point of 4D space-time at the origin of the universe (call it the origin point) is qualitatively different to the single point of 0D space-time (call it the nothing point) characterised by absolute nothingness, even though the two may appear to be very similar.
Analogy
Let's start with an intuitive visualisation of the difference by an analogy to a ball on a pool table.
Analogy
Let's start with an intuitive visualisation of the difference by an analogy to a ball on a pool table.
Consider two apparently identical pictures such as the one above, each showing a ball. If I tell you that one shows a ball in motion, while the other shows a stationary ball, you would be hard-pressed to tell the difference. Indeed, the ancient Greeks proposed a paradox suggesting that an arrow in flight cannot possibly move, essentially because it is stationary at each individual point in time.
The reason a ball in motion is different to a stationary ball is because it has a hidden property that cannot be detected if we can only inspect a single point in time. That property is called momentum.
The nothing point would be analogous to a stationary ball, while a ball in motion would represent the origin point.
The reason a ball in motion is different to a stationary ball is because it has a hidden property that cannot be detected if we can only inspect a single point in time. That property is called momentum.
The nothing point would be analogous to a stationary ball, while a ball in motion would represent the origin point.
This helps to visualise how although the nothing point and the origin point may appear to be similar superficially, this similarity ultimately proves to be an illusion. I would argue that the 4D point has a hidden property analogous to momentum that we can only see as time flows, and this property is its single dimension of time which provides an "arrow of time" pointing to the future.
The difference is because the nothing point is both a spatial and temporal singularity because it has no time dimension. At the origin point, only space is compressed into a singularity. They look alike due to the effective absence of space, but only in freeze-frame. The origin point has "momentum" due to the time dimension that the nothing point lacks.
Unlike the origin point, therefore, the nothing point has no timeline extending from it. Thus, there would in effect be no time and so nothing could ever change.
A confusing contradiction
Carrier seems to understand and perhaps even agree with all this, and so I am left confused as to why he disagrees with me. Take the following extract for example.
Another way to think of it is that the universe does not begin at a state of nothing to the left of the first point of time. The universe begins at that first point of time.
[...] there will never be a single point of space-time. You can search the entire history of that universe and you will never find it. Of all the things that could happen, that wasn’t it. You will find an originating point of space-time. But that’s not the same thing. Because that point is part of a line and always has been. There was never a time when it wasn’t.
Thus, there was never a time when there was nothing.
This is my point precisely. If there was no time when there was no time, if there was no time when there was nothing, then P1 is obviously wrong, because P1 states "in the beginning there was absolutely nothing".
I have asked for a clarification of this apparent contradiction, but so far no helpful response has been forthcoming.
Update: Dr. Carrier has kindly resolved the contradiction. When he states "there was never a time when there was nothing", he is using my concept of nothing as he is replying to me. My concept of nothing states that there is no time. Dr. Carrier believes that logical necessity requires that at least a single instant of time must exist, so his concept of nothing in P1 is different. Thus, while P1 may or may not be true, but my concept of nothingness could never be true in Carrier's view.
Links to Kalam
Finally, I want to note that much of Carrier's argument relates to how time instantaneously comes into being as a complete timeline. Even though it may appear to us that it is gradually extruded from an initial point at a rate of one second per second, this is only our subjective view from within that timeline. Instead, the universe can be viewed as a single changeless four dimensional structure with time as just one of its dimensions.
This is interesting because it recalls the views I sought to express in my blog post refuting the Kalam cosmological argument. This post explains why I do not believe the universe had to have a beginning to its existence even if the timeline within it does have a definite start, much as the prime numbers "begin" with the number two even though this sequence has existed eternally. If we look at it this way, the universe is never created. And if it is not created, we do not need to explain how it came to be created. Both Carrier's argument and the Kalam cosmological argument are moot.
Be that as it may, Carrier's way of looking at the universe seems to be very close to my own, which makes our disagreement all the more vexing.
Perhaps our points of view have more in common than might initially be apparent. Perhaps, in some way we may both be right. We may ultimately have the similar ideas but merely express them differently. In my next post, I will see if any compromise is possible between our two positions.
I have asked for a clarification of this apparent contradiction, but so far no helpful response has been forthcoming.
Update: Dr. Carrier has kindly resolved the contradiction. When he states "there was never a time when there was nothing", he is using my concept of nothing as he is replying to me. My concept of nothing states that there is no time. Dr. Carrier believes that logical necessity requires that at least a single instant of time must exist, so his concept of nothing in P1 is different. Thus, while P1 may or may not be true, but my concept of nothingness could never be true in Carrier's view.
Links to Kalam
Finally, I want to note that much of Carrier's argument relates to how time instantaneously comes into being as a complete timeline. Even though it may appear to us that it is gradually extruded from an initial point at a rate of one second per second, this is only our subjective view from within that timeline. Instead, the universe can be viewed as a single changeless four dimensional structure with time as just one of its dimensions.
This is interesting because it recalls the views I sought to express in my blog post refuting the Kalam cosmological argument. This post explains why I do not believe the universe had to have a beginning to its existence even if the timeline within it does have a definite start, much as the prime numbers "begin" with the number two even though this sequence has existed eternally. If we look at it this way, the universe is never created. And if it is not created, we do not need to explain how it came to be created. Both Carrier's argument and the Kalam cosmological argument are moot.
Be that as it may, Carrier's way of looking at the universe seems to be very close to my own, which makes our disagreement all the more vexing.
Perhaps our points of view have more in common than might initially be apparent. Perhaps, in some way we may both be right. We may ultimately have the similar ideas but merely express them differently. In my next post, I will see if any compromise is possible between our two positions.

No comments:
Post a Comment